Miniworkshop on PDE
报告人:王治安(香港理工大学)
报告题目:Food chain and alarm-taxis: modeling and analysis
报告简介:In this talk, we shall introduce models: the food chain model with spatial effects (diffusion and prey-taxis) and alarm-taxis models. The former one describes the predator-prey dynamics in three trophic levels and the latter one introduces an anti-predation mechanism to the predator-prey interactions. We shall present some analytical results recently obtained.
报告人:李进开(华南师范大学)
报告题目:Global solvability of non-heat conducting full MHD in 1D
报告简介:In this talk, I will present some recent works concerning the global solvability with large initial data to the non-heat conducting full magnetohydrodynamical (MHD) system, with or without magnetic resistivity and in the presence of arbitrary vacuum, as long as the initial data is suitably regular. This talk is based on two joint works with Mingjie Li.
报告人:江飞(福州大学)
报告题目:On Stability and Instability of Gravity Driven Navier-Stokes-Korteweg Model in Two Dimensions
报告简介:Bresch-Desjardins-Gisclon-Sart have derived that the capillarity can slow down the growth rate of Rayleigh-Taylor (RT) instability in the capillary fluids based on the linearized two-dimensional (2D) Navier-Stokes-Korteweg equations in 2008. Motivated by their linear theory, we further investigate the nonlinear RT problem for the 2D incompressible case in a horizontally periodic slab domain with Navier boundary condition, and rigorously verify that the RT instability can be inhibited by capillarity under our 2D setting. More precisely, if the RT density profile 
 satisfies an additional stabilizing condition, then there is a threshold
satisfies an additional stabilizing condition, then there is a threshold 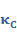
 of capillarity coefficient, such that if the capillarity coefficient
of capillarity coefficient, such that if the capillarity coefficient 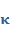
 is bigger than
is bigger than 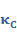
 , then the small perturbation solution around the RT equilibrium state is algebraically stable in time. In particular, if the RT density profile is linear, then the threshold
, then the small perturbation solution around the RT equilibrium state is algebraically stable in time. In particular, if the RT density profile is linear, then the threshold 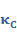
 can be given by the formula
can be given by the formula 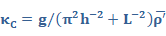
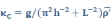 , where
, where 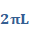
 denotes the length of a periodic cell of the slab domain in the horizontal direction, and
denotes the length of a periodic cell of the slab domain in the horizontal direction, and 
 the height of the slab domain. In addition, we also provide a nonlinear instability result for
the height of the slab domain. In addition, we also provide a nonlinear instability result for 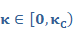
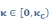 . The instability result presents that the capillarity can not inhibit the RT instability, if its strength is too small.
. The instability result presents that the capillarity can not inhibit the RT instability, if its strength is too small.
报告人:王志张(复旦大学)
报告题目:The geometric flow in Minkowski space
报告简介:In this talk, we study fully nonlinear curvature flows of noncompact spacelike hypersurfaces in Minkowski space. We prove that if the initial hypersurface is strictly convex and satisfies certain conditions, then the flow exists for all time. Moreover, we show that after rescaling the flow converges to a self-expander. We also will study the existence of self-expander with prescribed asymptotic behavior.
报告人:谢春景(上海交通大学)
报告题目:Liouville type theorem for steady Navier-Stokes system in a slab
报告简介:In this talk, we discuss some recent results on Liouville type theorems for steady Navier-Stokes system in a slab with either no-slip boundary conditions or periodic boundary conditions. In particular, we showed that bounded axisymmetric solutions in a slab must be zero or constant. The proofs are based on Saint-Vernant type estimate, which are quite elementary. This is a joint work with Jeaheang Bang, Changfeng Gui, and Yun Wang.
报告人:王焰金(厦门大学)
报告题目:Well-posedness of the Free Boundary Problem for the Euler-Fourier System
报告简介:We consider the free boundary problem for the Euler-Fourier system that describes the motion of compressible, inviscid and heat-conducting fluids. The effect of surface tension is neglected and there is no heat flux across the free surface. We establish the local well-posedness of the problem in Lagrangian coordinates under the Taylor sign condition. The temperature equation is regularized by a pseudo-parabolic equation so as to overcome the strong coupling effect between the Euler part and the Fourier part when constructing solutions. This is a joint work with Xumin Gu (Shanghai University of Finance and Economics).
报告人:耿世锋(湘潭大学)
报告题目:L1-convergence of solutions for compressible Euler equations with damping
报告简介:In this talk, the large time behavior of entropy solution to the compressible Euler equations for polytropic gas (the pressure 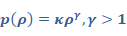
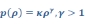 ) with damping is investigated. By introducing an elaborate iterative method and using the intensive entropy analysis, it is proved that the
) with damping is investigated. By introducing an elaborate iterative method and using the intensive entropy analysis, it is proved that the 
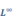 entropy solution of compressible Euler equations with finite initial mass converges strongly in the natural
entropy solution of compressible Euler equations with finite initial mass converges strongly in the natural 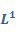
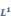 topology to a fundamental solution of porous media equation (PME), called by Barenblatt solution. We get better
topology to a fundamental solution of porous media equation (PME), called by Barenblatt solution. We get better 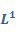
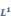 -convergence rate for
-convergence rate for 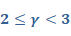
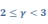 . We further prove the
. We further prove the 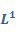
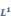 convergence to the Barrenblatt’s solution for any large
convergence to the Barrenblatt’s solution for any large 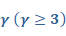
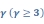 .
.
报告时间:
13日上午
时间 |
报告人 |
主持人 |
8:45-9:30 |
王治安教授(香港理工大学) |
屈长征教授(宁波大学) |
9:30-10:15 |
李进开教授(华南师范大学) |
屈长征教授(宁波大学) |
10:30-11:15 |
江飞教授(福州大学) |
耿俊教授(兰州大学) |
11:15-12:00 |
王志张教授(复旦大学) |
耿俊教授(兰州大学) |
14日上午:
时间 |
报告人 |
主持人 |
9:00-9:45 |
谢春景教授(上海交通大学) |
姚正安教授(中山大学) |
9:45-10:30 |
王焰金教授(厦门大学) |
姚正安教授(中山大学) |
10:45-11:30 |
耿世锋教授(湘潭大学) |
胡耀华教授(深圳大学) |
报告地点:汇星楼3号报告厅
欢迎感兴趣的师生参加!
菲律宾环球360注册账号
2023年2月11日